Recently, the research group led by Professor Li You from the Department of Physics in Tsinghua University has implemented a novel precision measurement technique in an atomic Bose-Einstein condensate. They demonstrated its potential application in microwave magnetic field sensing with a spatial resolution of several micrometers. The research findings have been published in Nature Physics under the title "Quantum enhanced sensing by echoing spin-nematic squeezing in atomic Bose-Einstein condensate".
In quantum technology, the use of superposition states inevitably introduces projection noise during measurements. Employing entangled particles for detection can reduce projection noise along a specific direction, thereby enhancing the signal-to-noise ratio. One of the most prominent examples is the spin squeezed state in a spin-1/2 system. This kind of multipartite entangled state can be visualized on the surface of Bloch sphere, spanned by the collective spin operators Jx, Jy, Jz.
The hyperfine ground state of rubidium-87 ultracold atoms can be regarded as a spin-1 system, which possesses a richer structure compared to the spin-1/2 system. Depending on the relative strength of the quadratic Zeeman energy shift (q) and the spin-exchange interaction strength (c2), the ground state of the spin-1 system may display three different phases: Polar (P), Broken-axis symmetry (BA), and Twin-Fock (TF) phases, separated by two quantum phase transition points at q=±2|c2|. Quenching through the phase transition points can generate entangled states that are useful for quantum precision measurements. During short-time spin-exchange dynamics, the number of atom pairs in the m=±1 states remains significantly lower than that in the m=0 state. The resulting quantum state is referred to as a two-mode squeezed vacuum, also known as a spin-nematic squeezed state. Analogous to the spin-squeezed state, the spin-nematic squeezed state can be intuitively represented on the spin-nematic Bloch sphere (Figure 1b).
Applying multipartite entangled states to quantum enhanced measurements often requires the utilization of interferometers. An interferometer is composed of path splitting, phase accumulation, and path recombining processes. If both the path splitting and recombination parts consist of nonlinear dynamics, the interferometer is referred to as a nonlinear interferometer. Utilizing entangled states in the phase accumulation process theoretically allows achieving precision beyond classical limit. However, due to experimental limitations, especially in terms of atom number detection resolution, observing significant quantum enhancements remains challenging.
Previous studies have indicated that when the path splitting and recombing parts of a nonlinear interferometer are time-reversed with each other, the constraints of detection noise can be overcome. However, implementing time-reversal in large systems is extremely difficult. In view of this, the research work presents a novel approach that achieves effective time reversal using spin-nematic squeezed echoes in a spinor Bose-Einstein condensate. Specifically, the researchers found that an effective time reversal can be realized by rotating the state to exchange the squeezing and anti-squeezing, after preparing the spin-nematic squeezed state (Figure 1c-f). This approach is efficient in overcoming the challenge of implementing time reversal in large systems, thereby enabling the utilization of spin-nematic squeezed echoes in interferometers for enhanced precision measurements.
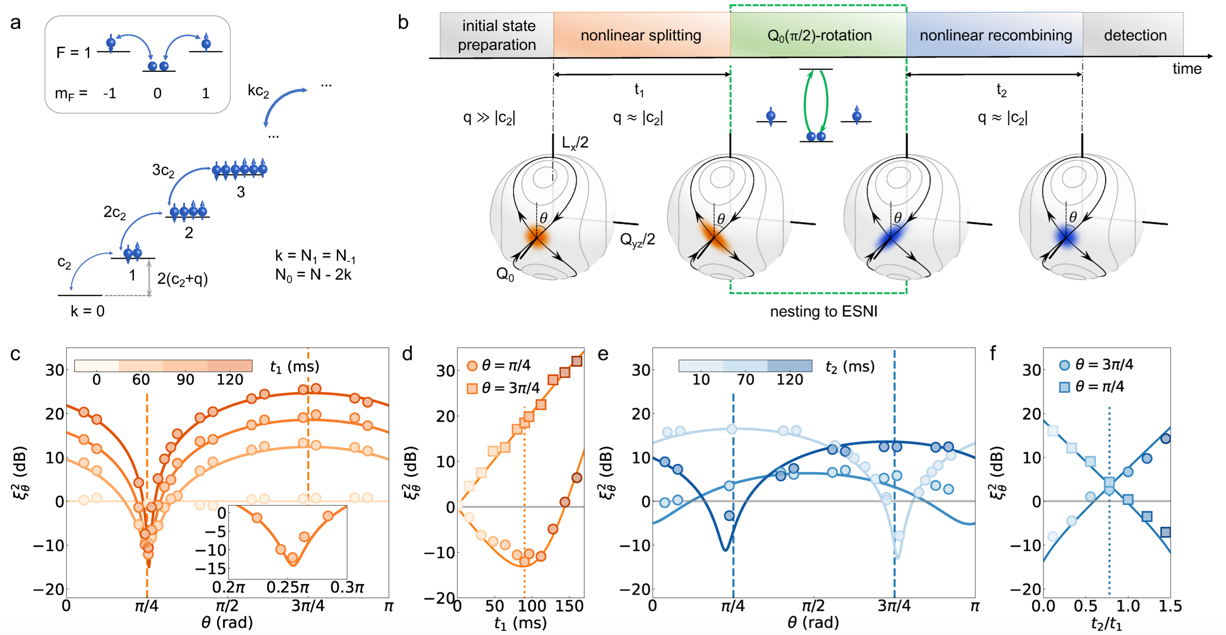
Figure 1. a, Structure of energy level in spin-1 atomic ensembles. b, schematic representation of effective time-reversal using state rotation. c-f, generation of spin-nematic squeezing and its effective time-reversal.
To quantitatively characterize the measurement precision of the interferometer, the researchers employed radio-frequency coupling to induce small-angle rotations in the experiments. By measuring the population of atoms in the m=0 state and utilizing error propagation formulas, they achieved a record sensitivity of 15.6±0.5 dB beyond the standard quantum limit (Figure 2). In comparison to linear readout results (11.7±0.3 dB), this method effectively mitigated the impact of detection noise.
Finally, the research group applied this nonlinear interferometer to the detection of nearly resonant microwave fields, achieving a sensitivity enhancement of 16.6±1.1 dB beyond the standard quantum limit. This enhancement surpassed the previous record set by the MIT group in 2022 using thermal atoms (12 dB) and currently represents the highest benchmark in quantum interferometric phase measurements.
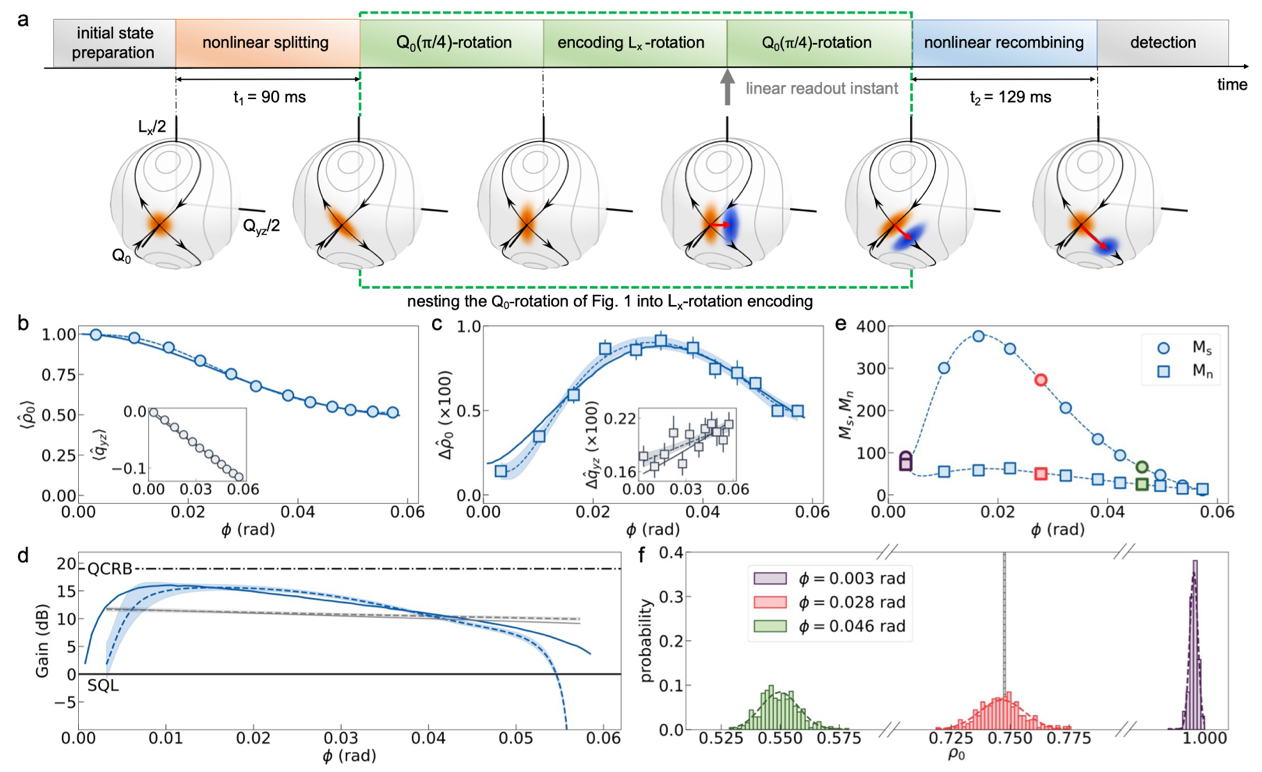
Figure 2. a, schematic of echo-based spin-nematic interferometry. b-e, dependence of averaged fractional population in m=0 state, standard deviation of population in m=0 state, metrological gain, signal and noise amplification factors on rotation angle. f, probability distributions of population in the m=0 state under various rotation angles.
This research lays the foundation for precision measurement applications based on ultracold atoms. The approach of achieving time-reversal through echo dynamics can be directly extended to systems with similar phase space structures, such as the Lipkin-Meshkov-Glick (LMG) model. It provides a new avenue for implementing nonlinear interferometers in other spin systems, offering innovative possibilities for advancing the field of precision measurements in diverse contexts.
The co-first authors of the research paper are, Dr. Tian-wei Mao, a Ph.D student since 2017, and Dr. Qi Liu, who defended Ph.D in 2021 in Tsinghua University. Qi Liu, Professor Yi-xiao Huang from Zhejiang University of Science and Technology, and Professor Li You from Tsinghua University are the corresponding authors. The research was supported by the National Natural Science Foundation of China, the Natural Science Foundation of Zhejiang Province, the Key Area Research and Development Program of GuangDong Province, the State Key Laboratory of Low-Dimensional Quantum Physics at Tsinghua University, and the Frontier Science Center for Quantum Information.
Link: https://www.nature.com/articles/s41567-023-02168-3
Editor: Li Han
`

